Get summaries, questions, answers, solutions, notes, extras, PDF and guide of Class 11 (first year) Economics textbook, chapter 7 Concepts of Revenue which is part of the syllabus of students studying under AHSEC/ASSEB (Assam Board). These solutions, however, should only be treated as references and can be modified/changed.
If you notice any errors in the notes, please mention them in the comments
Summary
The chapter focuses on different revenue concepts in microeconomics, particularly total revenue, average revenue, and marginal revenue. It starts by explaining that revenue is the money a firm receives from selling its product. The total revenue (TR) is calculated by multiplying the quantity of goods sold by the price per unit. For instance, if a firm sells 10 units of a product at ₹200 each, the total revenue is ₹2000.
Average revenue (AR) is the revenue per unit, calculated by dividing total revenue by the number of units sold. In most cases, average revenue is the same as the price, except when there is price discrimination. For example, if a firm sells different units at varying prices, AR might differ from the price per unit.
Marginal revenue (MR) is the additional revenue generated from selling one more unit of a product. If a firm sells 10 units at ₹200 per unit, the total revenue is ₹2000. If they sell an 11th unit at ₹190, the total revenue becomes ₹2090, and the marginal revenue for the 11th unit is ₹90.
The chapter also explores the relationship between TR, AR, and MR under different pricing situations. When prices remain constant, as in a perfectly competitive market, total revenue increases at a steady rate with output. Both AR and MR are equal to the price. However, when prices change, as in a monopoly or imperfect competition, AR and MR decrease as output increases. Marginal revenue falls more quickly than average revenue because MR only accounts for the additional unit, while AR includes all units sold.
The chapter concludes by highlighting that total revenue continues to rise until marginal revenue becomes zero, after which any further increase in output leads to a decline in total revenue. This indicates that marginal revenue can even become negative if the firm reduces prices to sell more units, causing total revenue to decrease.
The chapter provides various examples and mathematical formulas to illustrate these relationships and helps in understanding the role of revenue in the decision-making of firms.

Video tutorial
Textbook solutions
Very Short Answer Type Questions
1. Define revenue.
Answer: Revenue refers to the amount received by a firm from the sale of a given quantity of a commodity in the market.
2. Define average revenue.
Answer: Average revenue is defined as the revenue per unit of output sold.
3. What is meant by marginal revenue?
Answer: Marginal revenue is the net addition to total revenue from the sale of an extra unit of an output.
4. Which concept of revenue is called price?
Answer: Average revenue is called price.
5. What is the relation between marginal revenue and average revenue for a price-taking firm?
Answer: AR and MR will be equal at all levels of output.
6. What are the shapes of marginal revenue and average revenue curve when price is variable?
Answer: AR and MR curves coincide in a horizontal straight line parallel to X-axis.
7. Fill in the blanks:
(i) TR = P(price) x Quantity sold
(ii) When price is constant, AR = MR (Marginal Revenue)
8. When can MR be zero or negative?
Answer: MR can be zero or negative. MR can be zero when TR remains same with rise in output. MR can be negative when TR falls with increase in output.
Short Answer Type Questions
1. What happens to average revenue when marginal revenue is: (i) greater than average revenue; (ii) equal to average revenue; (iii) less than average revenue?
Answer: (i) When MR > AR, AR rises. (ii) When MR = AR, AR is constant. (iii) When MR < AR, AR falls.
2. Draw in a single diagram the average revenue and marginal revenue curves of a firm which can sell any quantity of the good at a given price. Explain.
Answer: When the firm can sell any quantity at a given price, both the AR and MR curves are horizontal straight lines and coincide with each other. This is because the price remains constant, so the revenue from each additional unit sold (marginal revenue) is the same as the average revenue, which is the price.
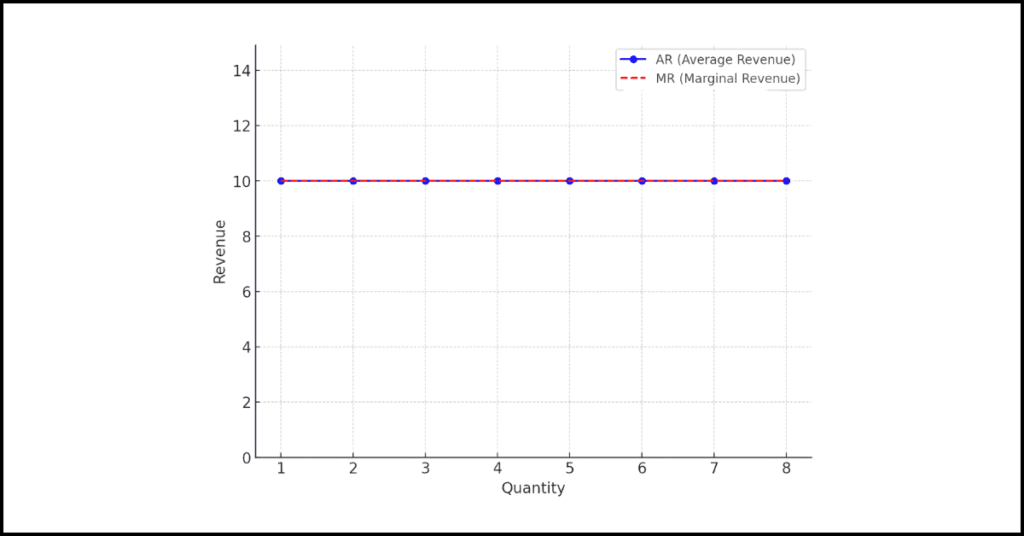
3. Draw average revenue and marginal revenue curves in a single diagram of a firm which can sell more units of a good only by lowering the price of that good. Explain.
Answer: When the firm can sell more only by lowering the price, the AR curve slopes downwards, indicating that average revenue falls as more units are sold. The MR curve lies below the AR curve, falling at a faster rate because each additional unit sold brings in less additional revenue than the average revenue.

4. What is revenue? Explain the relation between marginal revenue and average revenue.
Answer: Revenue is the income that a firm receives from the sale of goods and services.
Marginal revenue (MR) is the additional revenue gained from selling one more unit of a good, while average revenue (AR) is the revenue per unit sold, which is equal to the price. When MR is greater than AR, AR rises. When MR equals AR, AR remains constant. When MR is less than AR, AR falls.
5. Suppose the market price of each unit of a good is ₹5. A total of 6 units have been sold by a price-taking firm. Calculate TR, MR, and AR for the firm.
Answer: (i) Total Revenue (TR):
Total Revenue is calculated by multiplying the price (P) by the quantity sold (Q).
TR = P × Q
For each quantity:
- TR for 1 unit = ₹5 × 1 = ₹5
- TR for 2 units = ₹5 × 2 = ₹10
- TR for 3 units = ₹5 × 3 = ₹15
- TR for 4 units = ₹5 × 4 = ₹20
- TR for 5 units = ₹5 × 5 = ₹25
- TR for 6 units = ₹5 × 6 = ₹30
Thus, the TR values for the firm are:
- TR = ₹5, ₹10, ₹15, ₹20, ₹25, ₹30 for the respective quantities.
(ii) Marginal Revenue (MR):
Marginal Revenue is the additional revenue earned by selling one more unit. It is calculated as the difference between total revenue at the current level of output and total revenue at the previous level.
MR = TRn – TR(n-1)
For each quantity:
- MR for 1st unit = ₹5 (as there is no previous revenue)
- MR for 2nd unit = ₹10 – ₹5 = ₹5
- MR for 3rd unit = ₹15 – ₹10 = ₹5
- MR for 4th unit = ₹20 – ₹15 = ₹5
- MR for 5th unit = ₹25 – ₹20 = ₹5
- MR for 6th unit = ₹30 – ₹25 = ₹5
Thus, the MR values for the firm are:
- MR = ₹5 for each additional unit sold.
(iii) Average Revenue (AR):
Average Revenue is calculated by dividing the total revenue by the quantity sold.
AR = TR ÷ Q
For each quantity:
- AR for 1 unit = ₹5 ÷ 1 = ₹5
- AR for 2 units = ₹10 ÷ 2 = ₹5
- AR for 3 units = ₹15 ÷ 3 = ₹5
- AR for 4 units = ₹20 ÷ 4 = ₹5
- AR for 5 units = ₹25 ÷ 5 = ₹5
- AR for 6 units = ₹30 ÷ 6 = ₹5
Thus, the AR values for the firm are:
- AR = ₹5 for each unit.
6. When the average revenue curve of a firm is falling, marginal revenue curve lies below it? Why.
Answer: When the average revenue curve is falling, the marginal revenue curve lies below it because each additional unit sold brings in less revenue than the previous units. This is due to the necessity of lowering the price in order to sell more units, which reduces the additional revenue gained from each new unit sold.
7. When marginal revenue is zero, total revenue is maximum. Why? Explain.
Answer: Marginal revenue is the change in total revenue from selling one more unit. When marginal revenue becomes zero, it means that selling an additional unit does not change the total revenue, indicating that total revenue has reached its maximum. If marginal revenue becomes negative, total revenue would start to decrease. Therefore, total revenue is at its maximum when marginal revenue is zero.
Long Answer Type Questions
1. Explain the concepts of average revenue, marginal revenue and total revenue.
Answer: The amount spent by the consumer on the purchase of a commodity becomes revenue in the hands of the seller. There are three concepts of revenue: total revenue, average revenue, and marginal revenue.
Total Revenue (TR) refers to the total amount of money that a firm receives from the sale of its product, i.e., sale receipts. It depends upon a firm’s total sales. Total revenue is equal to the quantity sold multiplied by the selling price of the commodity, i.e., TR = Q × P, where P is the price per unit and Q is the total product sold.
Average Revenue (AR) is the revenue per unit of the commodity sold. It is found by dividing total revenue by the number of units sold. Thus, AR = TR ÷ Q. Since different units of a commodity are sold at the same price in the market, average revenue equals price.
Marginal Revenue (MR) is the net extra revenue obtained by selling an additional unit of the product. It is the addition made to the total revenue by selling one more unit of a commodity. Algebraically, it is the addition made to total revenue by selling n units of a product instead of (n-1), where n is any given number. Thus, MR = TRn – TR(n-1).
2. Explain how average revenue and marginal revenue are related to each other in terms of price elasticity of demand.
Answer: Average revenue and marginal revenue are related through the price elasticity of demand. The price elasticity of demand refers to how sensitive the quantity demanded of a good is to a change in its price.
- When the demand is perfectly elastic, the firm can sell any quantity at the same price. In such a situation, AR remains constant, and MR equals AR at all levels of output.
- When demand is less elastic, to sell more quantity, the firm must lower its price. In this case, MR will be less than AR, and both AR and MR will fall as the firm increases its output. The greater the fall in MR relative to AR, the less elastic the demand becomes.
- When MR is positive, AR decreases, but at a slower rate. When MR is negative, AR decreases at a faster rate. Therefore, the relationship between AR and MR can be used to infer the price elasticity of demand.
3. Explain the nature of AR and MR curves when price is fixed.
Answer: When the price is fixed, the AR and MR curves take on specific shapes.
- In a perfectly competitive market, where the firm is a price taker and the price is constant, the AR curve is a horizontal straight line, parallel to the x-axis, because AR equals the price at all levels of output.
- Since the price remains constant, the additional revenue from selling one more unit (MR) is also constant and equals the AR. Hence, the MR curve coincides with the AR curve and is a horizontal line parallel to the x-axis.
This shows that, under a fixed price situation, AR = MR = P, and both curves are straight and horizontal.
4. Explain the relationship between AR and MR when price is variable.
Answer: When the price is variable, the relationship between AR and MR changes. In this case:
- AR falls as the firm increases its output because, to sell more units, the firm must reduce the price of the product.
- MR falls faster than AR because MR refers to the additional revenue from selling one more unit, while AR refers to the average revenue from all units sold.
- The relationship between AR and MR can be summarized as follows:
- When AR is falling, MR is also falling, but at a greater rate.
- MR can become zero or negative, whereas AR cannot be zero or negative.
- MR will always lie below the AR curve.
This is because, as the firm lowers its price to sell more units, the loss in revenue from selling the previous units at a lower price affects MR more than AR.
5. Explain the relation among the TR, AR and MR of a monopoly firm with the help of an imaginary table and diagram.
Answer: In a monopoly market, the relation among Total Revenue (TR), Average Revenue (AR), and Marginal Revenue (MR) can be explained as follows:
- Total Revenue (TR): Total revenue increases as the firm increases its output. However, after reaching a certain point, it starts to decrease as more units are sold at a lower price.
- Average Revenue (AR): Average revenue represents the price per unit, and it falls as the firm increases its output because, in a monopoly, to sell more, the firm must lower its price.
- Marginal Revenue (MR): Marginal revenue decreases faster than AR because MR refers to the additional revenue gained from selling one more unit, which is affected by the drop in price for all previous units sold at a higher price.
The relationship between TR, AR, and MR is that as output increases:
- TR increases at first but eventually starts to decrease.
- AR continuously decreases as the firm lowers the price to sell more units.
- MR decreases more rapidly than AR and can become zero or negative.
Imaginary Table:
| Quantity (Q) | Price (P) | Total Revenue (TR = Q × P) | Average Revenue (AR = TR ÷ Q) | Marginal Revenue (MR = TRn – TRn-1) |
|---|---|---|---|---|
| 1 | 10 | 10 | 10 | 10 |
| 2 | 9 | 18 | 9 | 8 |
| 3 | 8 | 24 | 8 | 6 |
| 4 | 7 | 28 | 7 | 4 |
| 5 | 6 | 30 | 6 | 2 |
| 6 | 5 | 30 | 5 | 0 |
| 7 | 4 | 28 | 4 | -2 |
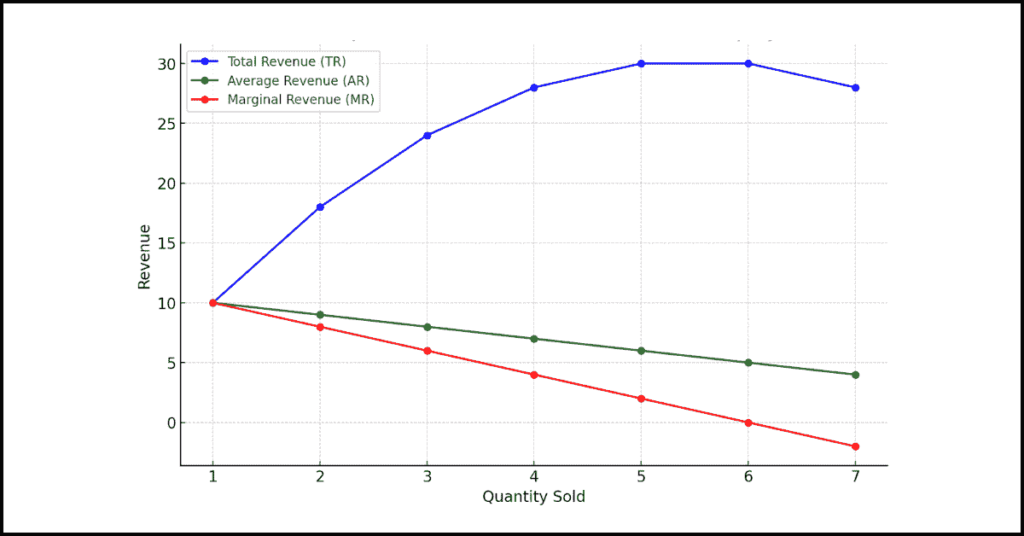
6. Suppose the price of a product under perfectly competitive market is 20. Calculate the total revenue (TR), marginal revenue (MR) and average revenue (AR) in the following table.
| Quantity (Q) | TR | MR | AR |
|---|---|---|---|
| 0 | |||
| 1 | |||
| 2 | |||
| 3 | |||
| 4 | |||
| 5 | |||
| 6 | |||
| 7 | |||
| 8 |
Answer:
| Quantity (Q) | TR | MR | AR |
|---|---|---|---|
| 0 | 0 | 0 | 0 |
| 1 | 20 | 20 | 20 |
| 2 | 40 | 20 | 20 |
| 3 | 60 | 20 | 20 |
| 4 | 80 | 20 | 20 |
| 5 | 100 | 20 | 20 |
| 6 | 120 | 20 | 20 |
| 7 | 140 | 20 | 20 |
| 8 | 160 | 20 | 20 |
(i) Calculation of Total Revenue (TR):
Total Revenue (TR) is calculated by multiplying the quantity sold (Q) by the price (P).
Given: Price (P) = 20 for all units.
| Quantity (Q) | TR (Q × P) |
|---|---|
| 0 | 0 × 20 = 0 |
| 1 | 1 × 20 = 20 |
| 2 | 2 × 20 = 40 |
| 3 | 3 × 20 = 60 |
| 4 | 4 × 20 = 80 |
| 5 | 5 × 20 = 100 |
| 6 | 6 × 20 = 120 |
| 7 | 7 × 20 = 140 |
| 8 | 8 × 20 = 160 |
(ii) Calculation of Marginal Revenue (MR):
Marginal Revenue (MR) is the additional revenue generated by selling one more unit.
MR for each quantity is calculated by the change in TR as quantity increases by one unit.
| Quantity (Q) | MR (TR at Qn – TR at Qn-1) |
|---|---|
| 0 | – |
| 1 | 20 – 0 = 20 |
| 2 | 40 – 20 = 20 |
| 3 | 60 – 40 = 20 |
| 4 | 80 – 60 = 20 |
| 5 | 100 – 80 = 20 |
| 6 | 120 – 100 = 20 |
| 7 | 140 – 120 = 20 |
| 8 | 160 – 140 = 20 |
(iii) Calculation of Average Revenue (AR):
Average Revenue (AR) is calculated by dividing the Total Revenue (TR) by the quantity (Q).
| Quantity (Q) | AR (TR ÷ Q) |
|---|---|
| 0 | – |
| 1 | 20 ÷ 1 = 20 |
| 2 | 40 ÷ 2 = 20 |
| 3 | 60 ÷ 3 = 20 |
| 4 | 80 ÷ 4 = 20 |
| 5 | 100 ÷ 5 = 20 |
| 6 | 120 ÷ 6 = 20 |
| 7 | 140 ÷ 7 = 20 |
| 8 | 160 ÷ 8 = 20 |
Extra
Additional MCQs
1. What is the formula for calculating Total Revenue (TR)?
A. TR = P x Q
B. TR = P + Q
C. TR = Q ÷ P
D. TR = P – Q
Answer: A. TR = P x Q
10. What happens to average revenue (AR) when marginal revenue (MR) is less than AR?
A. AR increases
B. AR stays constant
C. AR decreases
D. AR equals MR
Answer: C. AR decreases
Additional questions and answers
1. How is total revenue calculated?
Answer: Total revenue refers to the total amount of money that a firm receives from the sale of its product, i.e., sale receipts. It depends upon a firm’s total sales and can be expressed as a function of the total quantity sold.
Total revenue (TR) is calculated as TR = Q × P, where Q is the total quantity of product sold, and P is the price per unit.
For example, if a firm sells 10 units of a product at a price of ₹200 per unit, then the total revenue will be ₹2,000 (10 × 200).
14. Why does MR fall more rapidly than AR when output increases?
Answer: MR falls more rapidly than AR because MR is derived from only one additional unit of output, whereas AR is an average calculated over all units of output. This results in a comparatively lesser fall in AR than in MR. Thus, MR will always be below AR when the price decreases with an increase in output.

Get notes of other boards, classes, and subjects
